Lesson 3: Molecular Shape

Part b: Advanced VSEPR

Part 3a: Valence Shell Electron Pair Repulsion Theory (VSEPR)
Part 3b: Advanced VSEPR
Part 3c: Molecular Polarity
Part 3d: Hybridized Orbital Theory

 

Electron Pair Repulsions

The Valence Shell Electron Pair Repulsion Theory (VSEPR) is a model for thinking about how the valence shell electron pairs on an atom affect the way that those electrons and attached atoms arrange themselves in space. The model is built on the idea that electron pairs repel each other and spread into the space surrounding the central atom so as to minimize their repulsions. The three-dimensional orientation of the electron pairs is determined by these repulsions. The actual geometry assumed by the electron pairs is dependent upon the number of electron pairs or electron groups that surround the central atom. There is a subtle distinction between an electron pair and an electron group. A bond is considered one electron group regardless of whether it is a single bond (consisting of one electron pair), a double bond (two pairs), or a triple bond (three pairs).
 
In the previous part of Lesson 3, we discussed five different electron pair geometries. As a quick review, the connection between the number of repelling electron pairs or groups and the spatial distribution of those electron pairs about the central atom is pictured below.

 
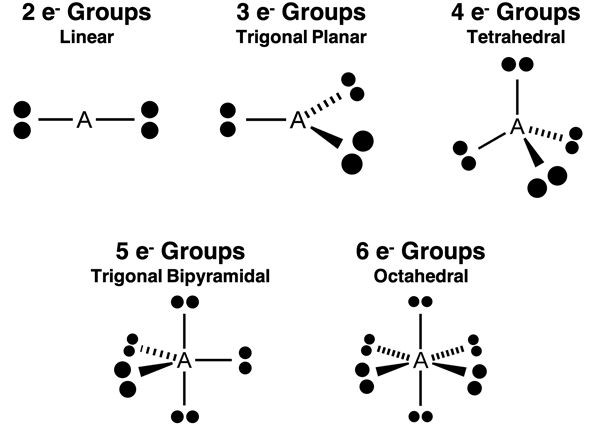
These are two-dimensional diagrams. In an attempt to represent the three-dimensional spatial relationships, the diagrams use solid lines, dashed lines, and wedged lines. A solid line can be perceived as being located in the plane of the screen. A wedged line comes out towards the viewer in front of the screen. A dashed line is extending into the space behind the screen and away from the viewer.
 
 
 

Determining Electron Pair Geometries

The first step to determining the electron pair geometry for a molecule is to construct the Lewis electron dot diagram. This MUST be done in order to determine the number of electron groups that surround the central atom. Once determined, you can use a table like the one below (or your memory) (or your spatial visualization skills) to associate the correct electron pair geometry with the number of electron groups. This table was presented on the previous page and discussed in great detail.

 
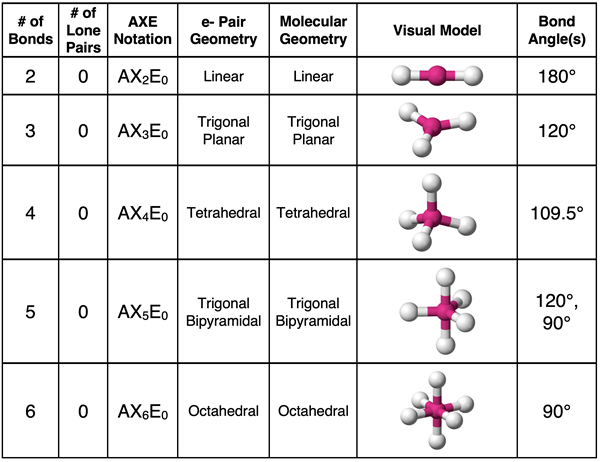
NOTE: We have discussed Lewis electron dot diagrams in an extensive manner beginning in Lesson 2b. Review Lesson 2b, Lesson 2c, and Lesson 2d if you need help with electron dot diagrams. We do not present details regarding such diagrams on this page.
 
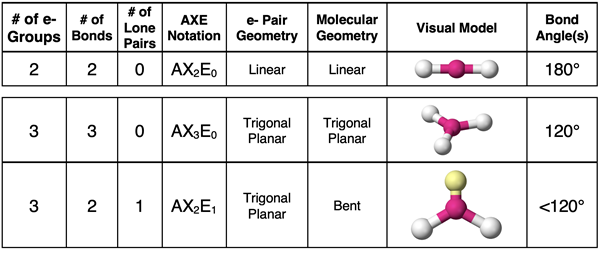
AXE notation is often used once the electron dot diagram is constructed. The notation, taking the form of AX_iE_j, indicates the number of bonds attached to the central atom (X_i where i is the number). The notation also indicates the number of lone pairs attached to the central atom (E_j where j is the number). The sum of i + j is the number of electron groups. The table below lists the various AXE notation possibilities for each of the five electron pair geometries.

 

 
 

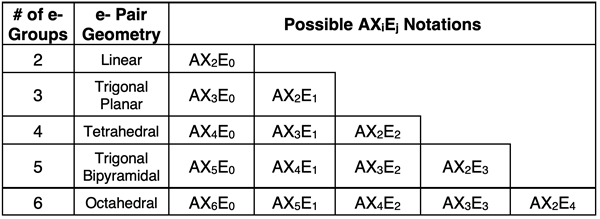
Molecular Geometry

Whether an electron pair is a lone pair or a bonding pair has no effect upon the actual electron pair geometry. All electron pair act similarly, repelling other electron pairs and competing for its share of space surrounding the central atom. The first page of this lesson was simplified by assuming all electron pairs were bonding pairs. The focus of this page is on the effect that lone pairs have upon molecular geometry.
 
Molecular geometry refers to the three-dimensional spatial arrangement of the terminal atoms of a molecule. The terminal atoms are connected to the central atom by the shared electron pair. They can be thought of as an extension of that electron pair. When all electron pairs are bonding pairs, the molecular geometry is identical to the electron pair geometry. But we know that sometimes the electron pair on a central atom is not shared with another atom. It simply sits upon the central atom as an electron pair.

 
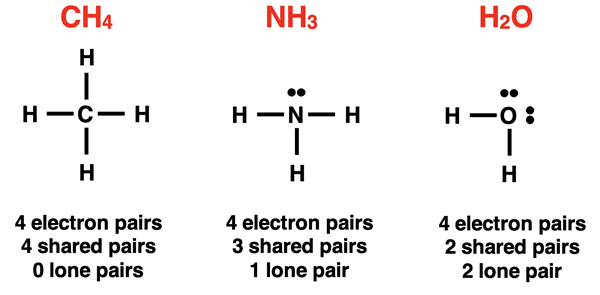
The presence of these lone pairs affects the molecular geometry because molecular geometry simply concerns itself with how the atoms are arranged. If an atom is removed and replaced with a lone pair, the molecular geometry will be affected. When describing the molecular geometry, we do not regard the lone pairs. The visual models below demonstrate how the name of the molecular geometry varies for three molecules with the same electron pair geometries.

 
In the remaining discussions, we will examine several sets of molecules having the same electron pair geometries but a varying number of lone pairs. The molecular geometry of each molecule in the set will be described.
 

 
 

Trigonal Planar Electron Pair Geometry

A molecule with three electron groups around the central atom will have a trigonal planar electron pair geometry about the central atom. If all electron groups are involved in bonding (no lone pairs), then the molecular geometry will also be trigonal planar. This is the case for a molecule like BF₃ shown below.
 
Now suppose that one of the electron groups surrounding the central atom is a lone pair and the others are bonding pairs. Such a molecule will be an AX₂E₁ molecule. The presence of the lone pair will result in a molecular geometry that is different than the electron pair geometry. The SO₂ molecule is an example. The molecular geometry is described as bent. “Bent” refers to the line drawn from the O atom to the central S atom to the second O atom. Dot diagrams and visual models for BF₃ and SO₂ are shown below.

 
 
 

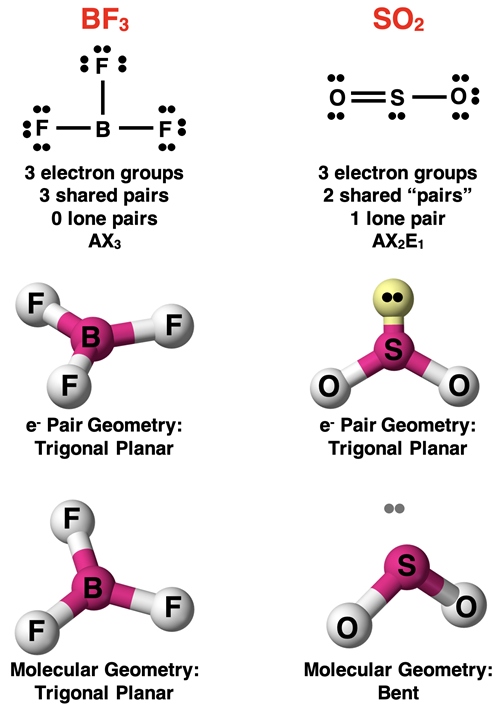
Tetrahedral Electron Pair Geometry

A molecule with four electron groups around the central atom will have a tetrahedral electron pair geometry. If all electron groups are involved in bonding (no lone pairs), then the molecular geometry will also be tetrahedral. This is the case for a molecule like CCl₄ shown below.

 
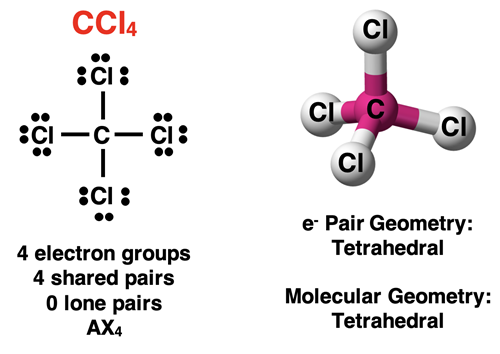
Molecules with four electron groups can have one lone pair or two lone pairs on the central atom. Let’s begin with the case of one lone pair and three bonding pairs. Such a molecule will be an AX₃E₁ molecule. The theme of this page will be that the presence of a lone pair will cause the molecular geometry to be different than the electron pair geometry. All AX₃E₁ molecules will have a molecular shape that is described as trigonal pyramidal. The three terminal atoms form a triangular base. The central atom is positioned above this base. Lines drawn up to the central atom will result in a pyramid. We observe a trigonal pyramidal shape for the PCl₃ molecule.

 
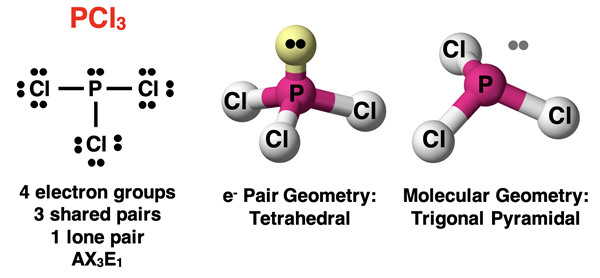
Now let’s suppose that two of the electron groups surrounding the central atom are lone pairs and the other two are bonding pairs. We would represent this as an AX₂E₂ molecule. An example would be H₂O or OCl₂. Once again, the lone pairs mean that the molecular geometry will not be the same as the electron pair geometry. These molecules will have a bent molecular geometry. A line drawn from the terminal atom to the central atom to the second terminal atom is a bent line.

 
NOTE: A final possibility for a tetrahedral electron pair geometry with lone pairs is an AX₁E₃ molecule. This is a molecule with one bonded atom and three lone pairs attached to the central atom. It is a two-atom molecule. The only shape any two-atom molecule can have is linear. That should not be too surprising, and it isn’t very exciting. We give little discussion on this page to two-atom molecules. They have a linear shape. Enough said.
 
 
 

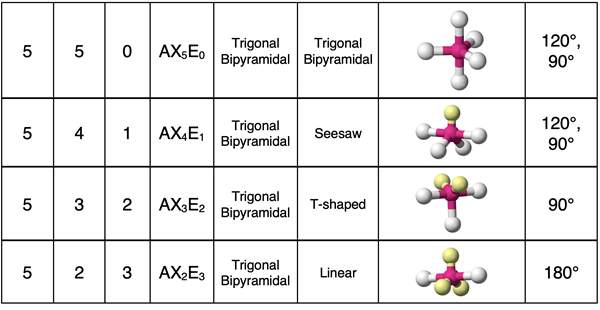
Trigonal Bipyramidal Electron Pair Geometry

A molecule with five electron groups around the central atom will have a trigonal bipyramidal electron pair geometry about the central atom. If all electron groups are involved in bonding (no lone pairs), then the molecular geometry will also be trigonal bipyramidal. This is the case for a molecule like PCl₅.

 
Molecules with five electron groups can have one, two, or three lone pairs surrounding the central atom. The molecular geometry gets a little tricky in these situations. There are five positions where atoms and lone pairs can be located – three in a plane and two above and below the plane. The three positions within the plane are referred to as the equatorial positions and the two positions above and below the plane are referred to as the axial positions. When lone pairs are present for this electron pair geometry, they will assume one of the three equatorial positions. Lone pairs tend to be drawn closer to the nucleus of the central atom since there are no terminal atoms pulling that lone pair away from the central atom. Because of this, lone pairs need a little extra room than the shared pairs. Since the equatorial positions are spaced apart by 120° angles, they offer more space for electron pairs. This explains why lone pairs prefer the equatorial positions.
 
Let’s begin with the case of one lone pair and four bonding pairs. Such a molecule will be an AX₄E₁ molecule. This lone pair will cause the molecular geometry to be different than the electron pair geometry. The lone pair occupies one of the three equatorial positions. The molecular geometry is described as seesaw. A line drawn from the atoms at the equatorial positions to the central atom from the legs of the seesaw. A line connecting the axial positions forms the beam or board of the seesaw. We observe a seesaw molecular geometry for the SF₄ molecule.

 
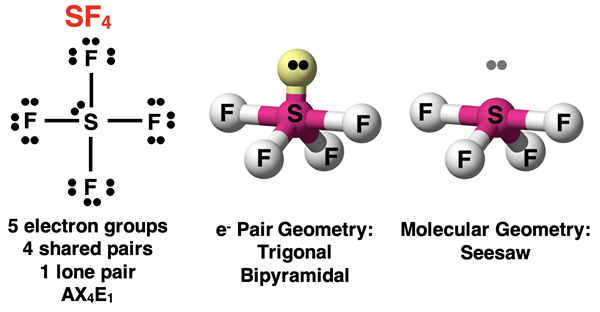
Now let’s consider the case of two lone pairs and three bonded atoms. Such a molecule will be an AX₃E₂ molecule. ClF₃ is an example of such a molecule. The lone pairs occupy two of the three equatorial positions. The third equatorial position and the two axial positions are occupied by atoms. This yields a molecular geometry that is referred to as T-shaped. A line drawn from the atom at the equatorial position to the central atom makes a right angle with a line drawn from the axial atom above the plane to the axial atom below the plane. These two lines form a T shape with each other.

 
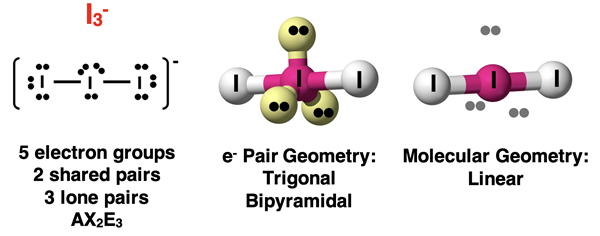
Finally, let’s consider three lone pairs and two bonded atoms. Such a molecule has an AX₂E₃ notation. The I₃^- ion is an example of this structure. The three lone pairs occupy the three equatorial positions. The two axial positions are occupied by the two atoms. The line connecting the axial atoms and the central atoms forms a molecular geometry that is referred to as linear. The Lewis electron dot diagram and the visual model are shown below.

 
 
 

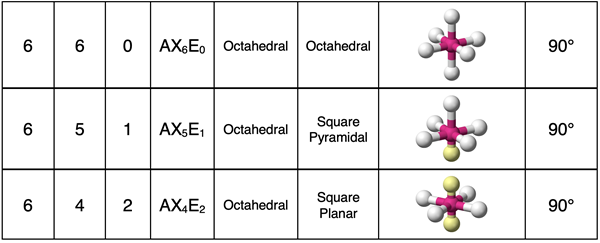
Octahedral Electron Pair Geometry

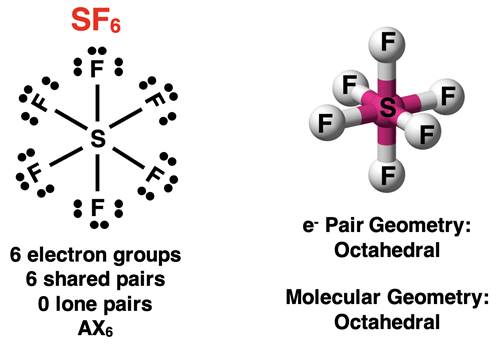
A molecule with six electron groups around the central atom will have an octahedral electron pair geometry about the central atom. If all electron groups are involved in bonding (no lone pairs), then the molecular geometry will also be octahedral. This is the case for a molecule like SF₆ shown below.

 
Let’s consider the case of one lone pair and five bonding pairs. Such a molecule will be an AX₅E₁ molecule. The ClF₅ molecule is an example. As always, the lone pair will cause the molecular geometry to differ from the electron pair geometry. Each position in the octahedron is equivalent. Unlike the trigonal bipyramidal electron pair geometry, there are no preferred locations for a lone pair. Put the lone pair at any location and then rotate the molecule so that is resembles the visual model in the middle part of the diagram below. Note that there are four atoms in the plane, one atom above the plane, and lone pair below the plane. The four atoms in the plane from a square. Including the atoms above the plane turns the square into a square pyramidal molecular geometry. The lone pair could be placed at any of the six possible locations of the octahedron and a 90° or 180° rotation of the octahedron will re-create the situation shown in the middle of the diagram below.

 
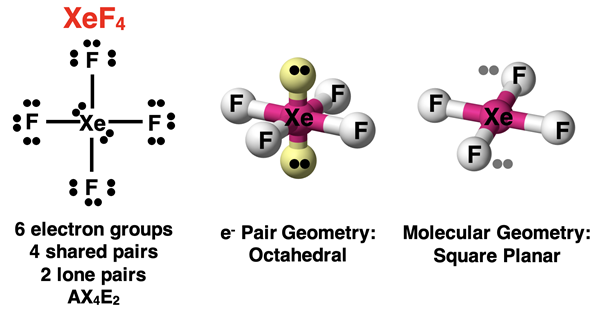
For our final example, consider the case of two lone pairs and four bonding pairs. This is an AX₄E₂ notation. An example would be XeF₄. As discussed earlier, the lone pairs need to have more space than the bonding pairs since they are pulled closer to the central atom. For this reason, the lone pairs need to be on opposites corners of the octahedron. It does not matter which opposite corners that they are on since all positions are equivalent. They just need to be opposite each other. (In the diagram, A and A’ are opposite each other. B and B’ are opposite each other. And so forth.) That leaves the four terminal atoms in a square plane with the central atom at its center. We refer to this molecular geometry as square planar.
 

 
 
 

Summary of Various Molecular Geometries

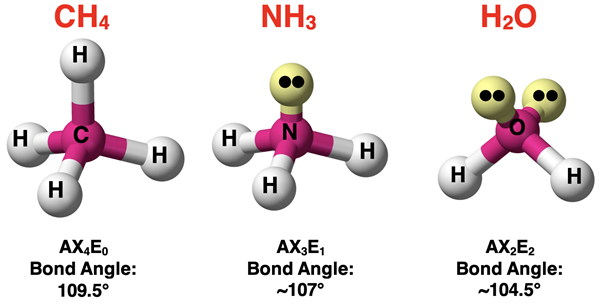
There is a lot of information and logic on this page. Electron dot diagrams, AX_iE_j notation, visual models with atoms and lone pairs, etc. It takes some time, some review, and lots of thinking and visualizing to digest the relationships between the number of electron groups, the number of lone pairs, and the molecular geometry. To assist in the process, we have provided the summary table below.

 
 
 
Lone Pairs and Bond Angles
The central atom and the terminal atoms form bonds with one another. As shown in the second column of the table, there are often two or more bonds. Picture the bonds as forming lines and intersecting with each other. The bond angle refers to the angle that these bond lines make with one another. The actual angle values are the result of geometric principles. But particularly for the rows showing tetrahedral electron pair geometry, you will notice that the angles decrease with additional lone pairs. The angles change from 109.5° to approximately 107° (one lone pair) to approximately 104.5° (two lone pairs). Every lone pair is pulled a bit closer to the nucleus of a central atom than a shared pair. This closer proximity to the central atom means the lone pair will need a little extra room and nudges the terminal atoms closer to one another. This causes the angle for AX₃E₁ to be slightly less than the angle for AX₄. And by similar logic, two lone pairs need even more room. And so, the bond angle for AX₂E₂ is a bit less than the angle for AX₃E₁.
 

 
 
 
 

Before You Leave

• Download our Study Card on Molecular Geometry. Save it to a safe location and use it as a review tool. 
• Practice! Try our Concept Builder titled Which One Doesn’t Belong – VSEPR Theory
• The Check Your Understanding section below include questions with answers and explanations. It provides a great chance to self-assess your understanding.

 
 
 

Check Your Understanding

Use the following questions to assess your understanding. Tap the Check Answer buttons when ready.
 
1. Which method best explains how to determine the AXE notation of a compound?

1. Look at the formula. You can always tell the AXE notation from CH₄ or NCl₃ or SO₃.
2. Draw the electron dot diagram. Then inspect the central atom for bonds and lone pairs.
3. Take a wild guess at it. It’s a lot easier. And when you get it right, it’s exhilarating.
4. Get the phone number of the smartest person in the class. Give them a call. Just ask.

 


 
2. TRUE  or  FALSE:
BCl₃ has a molecular geometry that is trigonal planar. Thus, any other molecule with a similar formula – like NCl₃ or even SO₃ – will have the same trigonal planar molecular geometry.
 
​

 
3. Under what conditions will the electron pair geometry and the molecular geometry be different? Explain your answer.
  
​

 
 
4. The diagrams depict the central atom (A) of a molecule. In each case, identify the AXE notation, the electron pair geometry, and the molecular geometry.
   



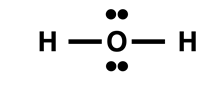
5. Two students are discussing the H₂O molecule. The electron dot diagram is shown. One student is claiming that the shape is linear. Convince the student why he is wrong.
 
​

 
 
6. For the following molecules (and ions), …

• Draw a Lewis electron dot diagram.
• Identify the AXE notation.
• Identify the electron pair geometry.
• Identify the molecular geometry.

 

1. BrF₅ 
   
   Check Answer
   Answer: 
   
   
   
    
   
    
2. XeCl₄ 
   
   Check Answer
   Answer: 
   
   
   
    
   
    
3. BrF₃ 
   
   Check Answer
   Answer: 
   
   
   
    
   
    
4. NO₂^- ion 
   
   Check Answer
   Answer: 
   
   
   
    
   
    
5. NCl₃ 
   
   Check Answer
   Answer: 
   
   
   
    
   
    
6. O₃ 
   
   Check Answer
   Answer: