Electricity: Electromagnetism

Electromagnetism: Problem Set Overview

We are planning 12 problem sets on the topic of Electromagnetism. Most problems are multi-part problems requiring an extensive analysis. The problems target your ability to apply concepts of elctric circuits and magnetic fields in order to analyze situations in which a moving charge induces a magnetic effect or a magnetic field induces a current in a wire.

 

The Origin of Magnetic Fields and Forces

Magnetic fields are produced by certain elements in nature. There are 4 levels of scale from smallest to largest that the element must satisfy for the object to display a magnetic field around it: 1) particle, 2) atom, 3) crystal structure, 4) domain.

1. Charged Particle:
   Electrons orbiting the nucleus are tiny magnets due to their motion and a quantum property called spin. It gives the particle a tiny magnetic field called a magnetic moment. In a broader sense, spin is an essential property influencing the ordering of electrons and nuclei in atoms and molecules. The spin state can be either up or down. Protons also have the magnetic property of spin, but it is about 1000 times less in magnitude compared to the electron’s spin.
    
2. Atom:
   Atoms have many electrons orbiting the nucleus that fill orbitals. Electrons in a specific orbital pair up and in general, each electron pair consists of an up and a down spin characterisitc that cancels out any overall magnetic effect. Therefore, atoms with half-filled outer shells have the best chance of being effectively magnetic.
    
3. Crystal Structure:
   Many elements form solids with atoms arranged in a crystal structure. This is defined as a particular repeating arrangement of atoms (molecules or ions) throughout the element. The atoms will arrange in a way that requires the lowest amount of energy, making it the most stable. In most of these arrangements the magnetic fields will be minimized.
    
4. Domain:
   Materials are made of sections of atoms with magnetic fields aligned. These sections are called domains. When one particular domain direction dominates the material, it will exhibit the physical characteristic of magnetism on a large scale.

Analyzing the periodic table and considering these 4 conditions, only iron, cobalt, and nickel are naturally occuring magnets at room temperature.

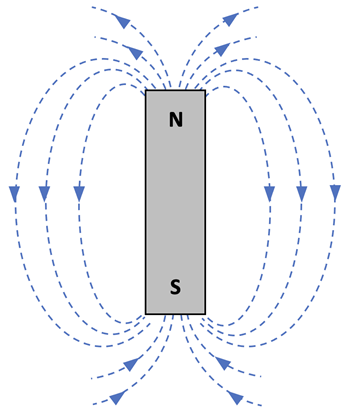
Any object able to create a magnetic field has polarity, producing north and south poles. Bar magnets create magnetic fields by having opposite poles (north and south) as shown at right. The direction of the field is the direction the north pole of a compass needle points at that location.

If 2 poles are sufficiently small and far apart, they can be considered point magnetic charges. The force between 2 magnetic points is much like the Coulomb Force Law between charges and Newton’s Universal Law of Gravity between masses: and is the permeability of the intervening medium.

 

The Magnetic Field Definition due to a Magnetic Force

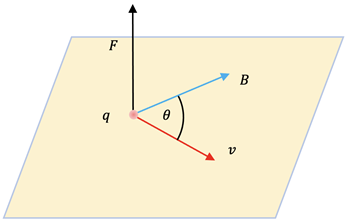It is found experimentally that the strength of the magnetic force on a moving charged particle is proportional to the magnitude of the charge, q , the magnitude of the velocity v, the strength of the external magnetic field, B, and the sine of the angle, Θ, between the direction of v and B as shown at right. This can be written as a cross product between v and B:

This expression is rearranged to define the magnitude of the magnetic field as the following:

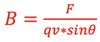
SI unit: Tesla

The direction of the magnetic field is determined by the right-hand rule as shown in the diagram.



 

The Magnetic Force on a Current in a Wire

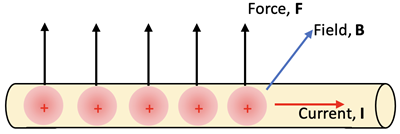The magnitude of the force from a magnetic field on a current in a wire can be analyzed by rewriting the equation for Force from the reference frame of a point in the wire where L is the length of the wire, Δq/Δt is the charge flow and B is the magnetic field as shown at right as follows:

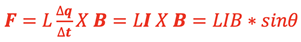
where I is the current in the wire and the direction is determined by the right-hand rule between conventional current, I, and magnetic field, B.

 

 

The Magnetic Force on the Current in a Loop

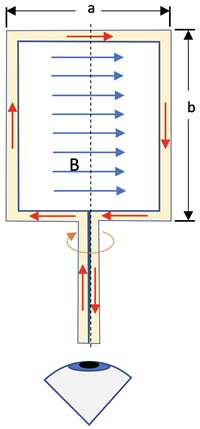The previous result can be used to analyze the force on a current in the shape of a rectangular loop as shown at right.

The current in the top and bottom sides of the loop is parallel to the B-field so the force from  

F = a*I X B = a*I•*B*sin(0°) = 0

The current in the left and right sides of the loop is perpendicular to the B-field so the result from F = b*I X B is

 F = b*I•*B*sin(90°) = b*I*B

The direction is obtained from the right-hand rule for cross products. The resulting forces are shown below from the eye’s view looking at the edge of the loop from the end position:

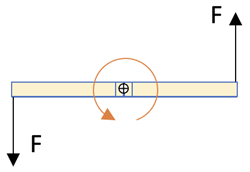
Since the forces do not go through the center of the loop a torque is produced that causes the loop to rotate about the center axis. The edge view reveals that the angle between the plane of the loop and the B-field, α, changes over time as shown below right.

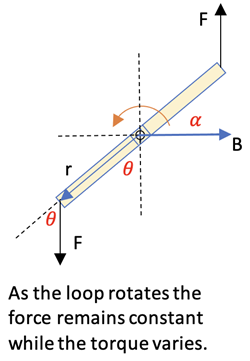The torque at any loop position about the center axis due to one force will be τ = r X F. Since r = a/2, F = b*I*B from above, and there are 2 identical forces, the net torque will be  

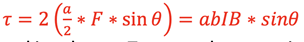
When the loop is horizontal Θ = 90°, making the net Torque value a maximum of τ_max = a*b*I*B.






 

A Magnetic Force Outcome; Hall Effect

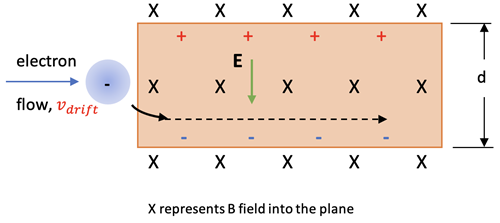When a flow of electrons moves through a wire in a magnetic field the charges are separated in the wire by the right-hand rule of F = q*v X B, where v is the drift velocity shown at right. This force on the electrons moves the negative charges to the bottom leaving positive charges near the top. This charge separation then sets up an E-field directed downward (called the Hall field) that puts a force on the moving electrons upward. Establishing equilbrium between these 2 forces prduces the following relationship:

F_net = 0 = q*E_Hall - q*v_drift*B

and rearranging gives

E_Hall = v_drift*B

From previous studies regarding separation of charge due to parallel plates the Potential Difference across the wire can be calculated by using ΔV = E*d. In this case, that Potential Difference is called the Hall emf:

ε_Hall = E_Hall*d = v_drift*B*d

 

 

A Magnetic Force Outcome; Mass Spectrometry:

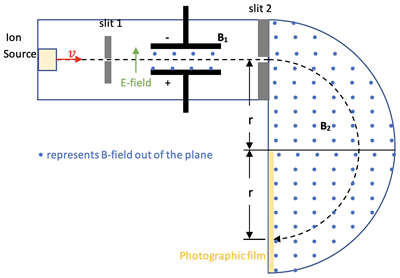A flow of ions can be accelerated to a velocity, v, and sent through a set of parallel plates as shown at right.  Opposing forces from the E-field and B-field are established with only ions having a certain velocity going straight through (F_net = 0) due to the relationship:

q*E = q*v*B₁

and rearranging gives

v = E / B₁.

These ions then enter another area having a magnetic field where they experince Uniform Circular Motion. Newton’s 2^nd Law gives the following result:

F = q*v*B₂ = m•v² / r

Rearranging gives

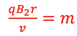
and substituting for v gives

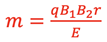
This result can be used to measure the mass of the ion.


 

The Magnetic Field Determined using Ampere’s Law

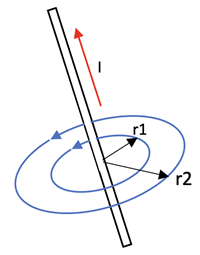In electrostatics a charge that feels a force in an electric field also produces an electric field. In the same way a moving charge in a wire that feels a force from a B-field also generates a B-field of its own. This B-field can be determined using calculus and Ampere’s Law for moving charges

The result for the current in the wire at right using Ampere’s Law is the following:

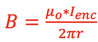
where µ_o = 4•π•10^-7 T*m/A

I_enclosed is is the current in the wire, and
 
r is the distance from the wire to the location of interest.


 

The Magnetic Field created by the Current in a Solenoid

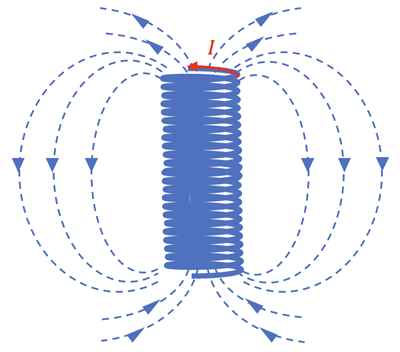A long coil of wire consisting of many loops is called a solenoid as shown at right. Applying the right-hand rule to determine B-field lines when a current is sent through the wire gives the result that the B-field is reinforced in the center of the coil and not reinforced between the individual coils or immediately outside the coils.
 
A solenoid acts like a bar magnet in that when current flows it has a north pole and a south pole, depending on the direction of the current. If the solenoid is very long in comparison to its diameter and the coils are tightly packed together, the field inside a solenoid is very nearly uniform in both direction and strength.






A solenoid cut in half with current shown in the coils is shown below.

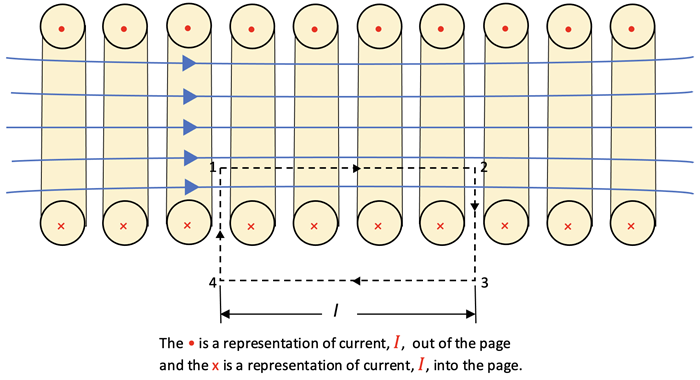
Ampere’s Law

can be used on a section of coils on one side of the solenoid as shown above using the dotted rectangle (N=4 loops) to determine the magnitude of the B-field. The closed surface must be analyzed on all sides. Sides 2-3 and 4-1 are perpendicular to the B-field so no contribution will occur due to the dot product giving a cos (90˚) = 0. Side 3-4 has such a small B-field due to canceling effects from all coils so it will not contribute. The only side needing to be evaluated is side 1-2. The result is the following:

B * L = µ_o * I_enc

where I_enc = N* I, 

µ_o = 4•π•10^-7 T*m/A, and
 
L is the length of the rectangle.
 
Therefore, the B-field produced by any solenoid can be calculated by using



where n = N / L, the number of loops per unit length.

 

The Magnetic Field created by the Current in a Toroid

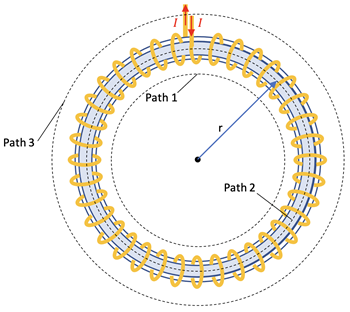A long coil of wire consisting of many loops bent into the shape of a circle is called a toroid as shown at right. Ampere’s Law

can be applied to the 3 pathways shown. Since there is no current inside Path 1 the B-field there is 0. Since the current flows into and out of the screen as shown at bottom right inside Path 3 the total current is 0 so the B-field there is 0.
 
Only in Path 2 do you get a current in one direction that qualifies for I_enc as part of Ampere’s Law. The result for Path 2 then will be the following:

B*2*π*r = µ_o * N * I

where N*I is the number of loops times the current.

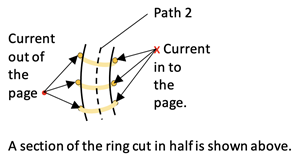The magnetic field is uniform within the toroid if the radius of the center of the ring, r, is large relative to the width of the ring. Solving for the B-field magnitude then gives
 

where  n = N / 2 π r, the number of loops per unit length.



 

 

The Magnetic Flux

In the 1800’s Michael Faraday used the setup above to perform the following experiments:

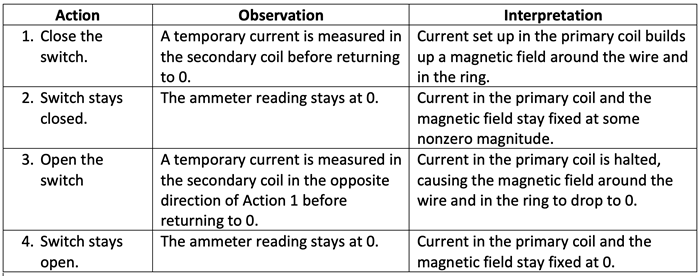
Because of the brief occurences of current in the secondary coil, it was concluded that a changing magnetic field in the ring produced an electric field in the secondary coil as though a source of potential difference [referred to as an induced emf (electromotive force)] was connected to it for a short period of time.
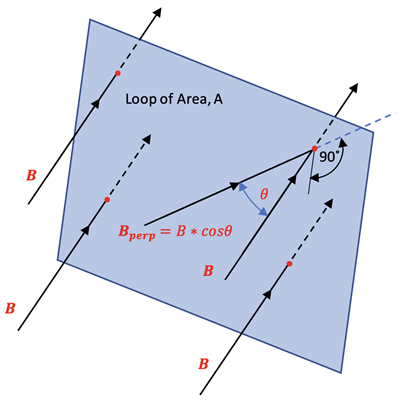
To study this phenomenon, the Magnetic Flux,  Φ_B, that is a measure of the strength of the magnetic field, B, passing through a specific cross-sectional area, A, is defined. It can be written as

Φ_B = B_{perpendicular} * A = B*A*cos Θ

SI unit: Weber (Wb)

B_{perpendicular}  is the component of B perpendicular to the plane of the loop as shown at right.






 

Faraday’s Law of Induction

It has been determined that an emf (electromotive force) is induced in a circuit whn the magnetic flux through the circuit changes with time. The relationship between the change in flux and the emf is referred to as Faraday’s law of Magnetic Induction and written as follows:

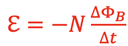
 where N would be the number of tightly wound loops that experience the change in magnetic flux, ΔΦ_B, during the time interval, Δt. The negative sign is used to determine the polarity of the induced emf. This is determined using Lenz’s Law stated as follows:

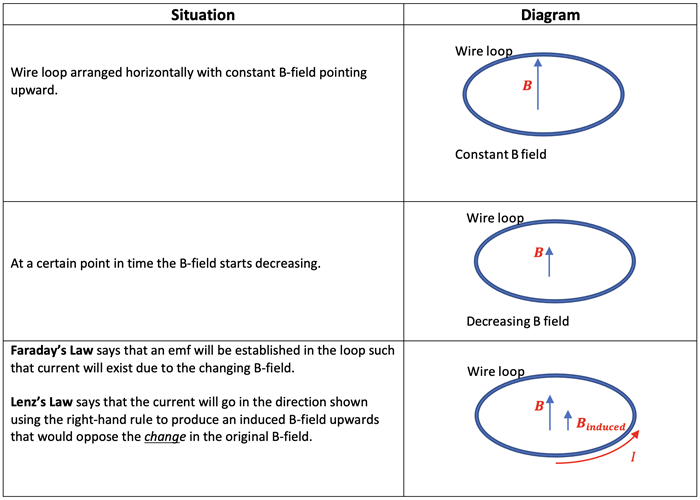
Lenz’s Law: The current caused by the induced emf travels in the direction that creates a magnetic field with flux opposing the change in the original flux through the circuit.
 

The creation of an emf due to a decreasing B-field is described in the table below:

The creation of an emf due to an increasing B-field is described in the table below:

The creation of an emf due to a moving conductor and/or changing area in a magnetic field is called Motional emf. Let’s look at 2 scenarios below.

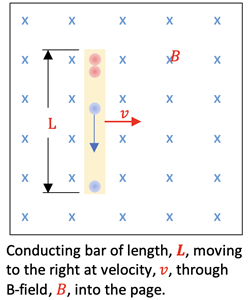Scenario 1:Moving conductor:
Conducting bar moving in a magnetic field as shown at right. A force due to the motion of charged particles is exerted on the charges in the bar, F = q*v X B, causing a separation of charge by forcing negative electrons to move to the bottom of the bar according to the right-hand rule. This sets up an electric field pointing downwards in the conductor. Charge stops moving in thhe bar when the force due to the electric field matches the opposing force due to the magnetic field. Therefore, F_e = F_B, and with substitution becomes q*E = q*v*B, giving E = v*B.

Like the capacitor, the potential difference (in this case the emf) set up by the separation of charge for the uniform electric field is E*L, so that the emf is the following:  

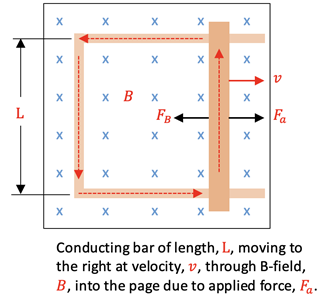Scenario 2: Changing Area:
Consider a conducting bar experiencing an applied force, F_a, to the right in a magnetic field as shown at right. A force due to the motion of charged particles is exerted on the charges in the bar, F = q*v X B, causing a counterclockwise conventional current in the closed conductive pathway by the dotted red lines. The continual movement of the charge upwards in the moving bar causes the bar to experience a force, F_B, to the left due to F = q*v X B, applying the right-hand rule.

Therefore, an induced emf will be set up to oppose this increasing area and can be calculated using a change in Magnetic Flux. Consider the change in area shown at right with the bar covering a distance, Δx,in a time of Δt. Using Faraday’s Law of Induction

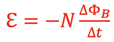
where N= 1 becomes



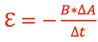
 and since ΔA = L*Δx,
 
   
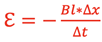
and since v = Δx/Δt, then the magnitude of the emf is: ε = B*L*v .
 

 
 

 

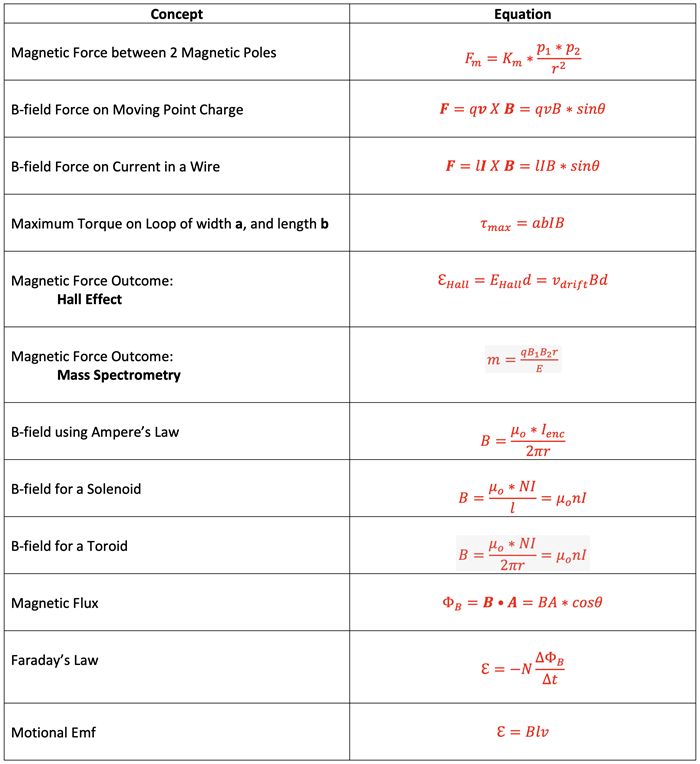
Equation Summary

 

Habits of an Effective Problem-Solver

An effective problem solver by habit approaches a physics problem in a manner that reflects a collection of disciplined habits. While not every effective problem solver employs the same approach, they all have habits which they share in common. These habits are described briefly here. Effective problem-solvers ...

• ...read the problem carefully and develops a mental picture of the physical situation. If needed, they sketch a simple diagram of the physical situation to help visualize it.
• ...identify the known and unknown quantities in an organized manner, often times recording them on the diagram itself. They equate given values to the symbols used to represent the corresponding quantity (e.g., F = 0.025 N, E = 4.50x10^-6 N/C, q = ???).
• ...plot a strategy for solving for the unknown quantity. The strategy will typically center around the use of physics equations and is heavily dependent upon an understanding of physics principles.
• ...identify the appropriate formula(s) to use, often times writing them down. Where needed, they perform the needed conversion of quantities into the proper unit.
• ...perform substitutions and algebraic manipulations in order to solve for the unknown quantity.

Read more...